
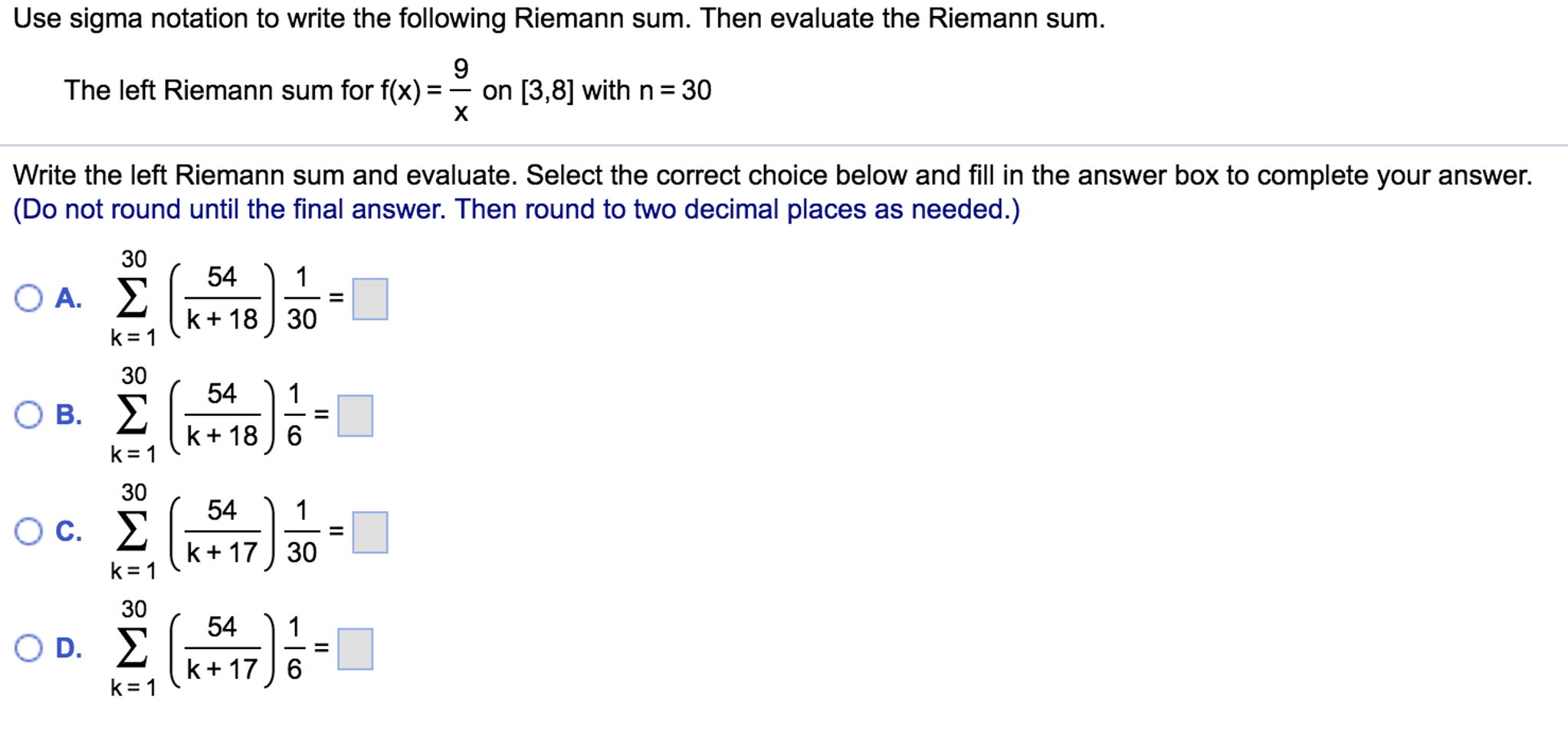
It really is just sort of a visual reminder that we are dealing with infinitesimal changes in x, that is, dx. As we make Δx smaller and smaller, until it is infinitesimal, we again change the notation from Δx to dx AND we change the notation of Σ to ∫, that is Σf(x)Δx to ∫f(x)dx. The Riemann sum is a sum of sections whose width is Δx, so we have, in general, Σf(x)Δx. It is pretty much the same deal on how we went from Σ to ∫. So for the slope of a straight line or secant line we used Δy/Δx but for the slope of a tangent line we use dy/dx. We decided that once the Δ of the Δy/Δx was infinitesimally small, we would change the notation from Δy/Δx to dy/dx, to remind us that we are dealing with infinitesimals (also called differentials). The we asked, what happens as Δy/Δx gets smaller and smaller, that is, the endpoints of the secant line get closer and closer to c. If we wanted to estimate the slope at a point c, we drew a line between two points on either side of c that were – Δy/Δx and Δy/Δx from c. The concept was developed by using a secant line, or average slope. When you got to differential calculus the problem was not the slope of a line, but the slope of a curve at some point. When you were in algebra you calculated the slope of a line as Δy/Δx. If you have made it to integral calculus, you must have come through algebra and differential calculus, and if so, you have already seen a change in notation, so I’ll start there. You have two questions here, one of notation, how did we go from Σ to ∫, and the other of how did definite integral come about. Could you get not just an approximation, but the exact area under the curve if you took the left side approximation and added it to the right side approximation and then divided that sum by 2? In other words, would the amount that you overestimated the area under the curve be exactly the same amount that you underestimated the area under the curve? Would the value of the overestimation (in either the left-side approximation, or right-side approximation) be equal to the value of the underestimation? My guess is that if the function has a vertical line of symmetry AND the interval along the x-axis extends an equal distance away from the line of symmetry in both directions (in our example -3 to 3 with the line of symmetry being the y-axis) that the amount of overestimation and underestimation would be the same and when you took the average of the two amounts you would be left with the exact value of the area under the curve. Summation Formula: The sum of sigma notation can be written as Σ_ $.Let's say you have a function y=x^2 and you want to find the area under the curve along the interval -3 to 3 and you're delta x was 6 (or any finite number). Question: Riemann sums in summation notation You might need: Calculator The following graph shows a Riemann sum: f(x)=x+4 2 1.5 0.5+ a म 3 Which of the following … Limit of summation calculator - Math Online. Find the riemann sum in sigma notation Answer: Step (i): Calculate the width The whole length is divided into 3 equal parts, x i = 0 and x l = 3, Width of an … Solved Riemann sums in summation notation You might need. WikiZero Özgür Ansiklopedi - Wikipedia Okumanın En Kolay Yolu Riemann Sum - GeeksforGeeks. Riemann sum summation notation calculatorWikizero - Euler–Maclaurin formula.


 0 kommentar(er)
0 kommentar(er)
